Albert Einstein famously referred to compounding interest as the eighth wonder of the world. He went on to state that those who understand it, earn it and those who don’t, will pay it. It is therefore important to understand what interest is, where compounding interest fits in and how to use it in your everyday life.
Interest rates are the cost of borrowing money. If you are the participant lending out the money, you receive the interest. If you deposit money in your bank account, it is similar to “lending” money to the bank and therefore you receive interest on the amount you deposit.
Simple Interest
Interest only accrues on the principal amount that is invested or borrowed. Usually the interest will accrue annually, but it is important to understand the contract as the accrual may be more often than a year, such as monthly, quarterly or bi-annually.
Example:
R200 invested with an interest rate of 3% for 2 years (nothing is mentioned about how often the interest accrues; therefore, we assume it is annually).
Year 1: Interest: R200 x 3 /100 = R6
Year 2: Interest: R200 x 3 /100 = R6
Total interest over two years: R6 + R6 = R12
Compounding Interest
Interest is earned on the principal amount invested and on interest previously earned. Similar to what was mentioned at simple interest, we usually see interest accruing annually, but it can happen more often that annually.
Example:
R200 invested with an interest rate of 3% for 2 years (nothing is mentioned about how often the interest accrues; therefore, we assume it is annually).
Year 1: Interest: R200 x 3 /100 =R6
Year 2: Principal amount increased by interest earned in previous year: R200 + R6 = R206
Interest: R206 x 3 /100 = R6.18
Total interest over two years: R6 + R6.18 = R12.18
In the two examples above, it was assumed that interest compounds annually. Compounding means how often the interest is added onto the principal amount. This is especially important when we work with compounding interest because every time the interest is added onto the principal amount, interest is being calculated on a higher and higher principal amount. When we compare interest or when we do interest calculation it is important to know how often during a year interest is being compounded. As mentioned, it can be annually, monthly, quarterly or bi-annually.
How would you calculate compounding interest that accrue monthly?
Example
You borrowed an amount of R5,000 at an annual interest rate of 5%, compounding monthly. What will the outstanding amount be that you need to repay at the end of 10 years?
A = P(1 + r/n) ^ (nt)
A = 5,000(1 + 0.05 /12) ^ (12×10)
A = 5,000(1 + 0.00416) ^ 120
A = 5,000(1.6470095)
A = 8,235.047
Thus, at the end of 10 years, you will have to repay a total of R8,235.05 (the principal of R5,000 plus the interest of R3,235.05).
Effective interest rate vs nominal interest rate
An interest rate takes two forms: nominal interest rate and effective interest rate. The nominal interest rate does not take into account the compounding period. The effective interest rate does take the compounding period into account and thus is a more accurate measure of interest charges.
This is important because you need to be able to compare apples with apples. The only way to do this is if we can compare the annual amount of interest that will be earned given the amount of times interest is compounded.
A statement that the “interest rate is 10%” means that interest is 10% per year, compounded annually. In this case, the nominal annual interest rate is 10%, and the effective annual interest rate is also 10%. However, if compounding is more frequent than once per year, then the effective interest rate will be greater than 10%. The more often compounding occurs, the higher the effective interest rate.
How to convert nominal rate to an effective rate to allow to make comparisons and to understand how much interest you will really pay or earn:
For clarification, n will be the same as m if we are just converting nominal interest rate to effective interest rate during a one-year period. If we need to consider more than one year, n will be equal to m multiplied by the number of years we consider.
Example:
A credit card company charges 21% interest per year, compounded monthly. What effective annual interest rate does the company charge?
r = 0.21 per year
m = 12 months per year (because we do not want to know how much interest needs to be paid over the period the loan is made, m and n is the same value.)
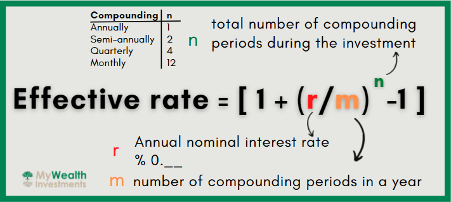
Effective rate = [ 1 + (r/ m)^n – 1 ]
Effective rate = [ 1 + (0.21/ 12)^12 – 1 ]
Effective rate = [1 + 0.0175 ]^12 – 1
Effective rate = (1.0175)^12 – 1 = 1.2314 – 1
Effective rate = 0.2314 = 23.14%
Thus, taking the compounding effect into account, the real amount of interest paid during a year is higher than only considering the nominal interest.
Read also:


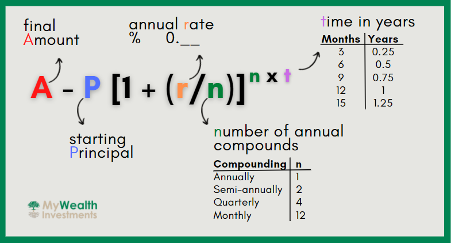





2 Comments
I was looking through some of your posts on this website
and I believe this web site is real informative! Retain putting up.Blog monetyze
To the regenesys.net webmaster, Your posts are always well received by the community.